國立政治大學113學年度學士班轉學生招生考試
考試科目:微積分(二)
系所別:應用數學系二年級
解答:$$\lim_{n\to \infty} \left({1\over \sqrt n\sqrt{n+1}} +{1\over \sqrt n\sqrt{n+2}} + \cdots {1\over \sqrt n\sqrt{n+n}}\right) =\lim_{n\to \infty} \sum_{k=1}^n {1\over \sqrt n\sqrt{n+k}}\\ =\lim_{n\to \infty} \sum_{k=1}^n {1\over \ n\sqrt{1+k/n}} =\int_0^1 {1\over \sqrt{1+x}}\,dx =\int_1^2 {1\over \sqrt u}\,du = \bbox[red, 2pt]{2(\sqrt 2-1)}$$解答:$$\textbf{a. }u=\sin x \Rightarrow du =\cos x\,dx \Rightarrow \int_0^{\pi/2} \cos x\sin(\sin x)\,dx = \int_0^1 \sin u\,du= \bbox[red, 2pt]{1-\cos 1} \\ \textbf{b. } \cases{u=\sec x\\ dv= \sec^2 x dx} \Rightarrow \cases{du =\sec x\tan x\,dx\\ v=\tan x} \Rightarrow I=\int \sec^3 x\,dx =\tan x\sec x-\int \sec x\tan^2 x\,dx \\= \tan x\sec x-\int \sec x(\sec^2-1) x\,dx =\tan x\sec x-I+ \int \sec x\,dx \\ \Rightarrow 2I=\tan x\sec x+\int \sec x\,dx =\tan x\sec x+\ln|\sec x+\tan x| \Rightarrow I={1\over 2}\left( \tan x\sec x+\ln|\sec x+\tan x| \right) \\ \Rightarrow \int_0^{\pi/4} \sec^3x \,dx = \left. \left[ {1\over 2}\left( \tan x\sec x+\ln|\sec x+\tan x| \right) \right] \right|_0^{\pi/4} = \bbox[red, 2pt]{{1\over 2}(1+\ln 2)}\\\textbf{c. } \cases{u=\ln x\\ dv=dx} \Rightarrow \cases{du={1\over x}dx\\ v=x}\Rightarrow \int \ln x\,dx = x\ln x- \int 1\,dx =x\ln x-x+c_1\\\quad \Rightarrow \int_0^1 \ln x\,dx = \left.\left[ x\ln x- x\right] \right|_0^1 = \bbox[red, 2pt]{-1}$$
解答:$$\cases{u=\sin^{n-1} x\\ dv= \sin xdx} \Rightarrow \cases{du= (n-1) \cos x\sin^{n-2}x\,dx \\ v=-\cos x} \\\Rightarrow I=\int \sin^n x\,dx = -\cos x\sin^{n-1}x +(n-1)\int \cos^2 x\sin^{n-2}x\,dx \\ \qquad = -\cos x\sin^{n-1}x +(n-1)\int (1-\sin^2x) \sin^{n-2}x\,dx\\ \qquad = -\cos x\sin^{n-1}x +(n-1)\int \sin^{n-2} x\,dx -(n-1)I \\ \Rightarrow nI=-\cos x\sin^{n-1}x +(n-1)\int \sin^{n-2} x\,dx\\ \Rightarrow I=-{1\over n}\cos x\sin^{n-1}x +{n-1\over n}\int \sin^{n-2} x\,dx ,\bbox[red, 2pt]{QED.}$$
解答:$$\textbf{a. } \int_{-\pi}^\pi \sin(mx)\cos(nx) \,dx = {1\over 2}\int_{-\pi}^\pi \left(\sin((m+n)x) +\sin((m-n)x) \right) \,dx \\= {1\over 2}\left(\left.\left[ -{1\over m+n}\cos((m+n)x) \right] \right|_{-\pi}^\pi +\left.\left[ {1\over m-n} \cos((m-n)x)\right] \right|_{-\pi}^\pi \right) =0+0=0, \bbox[red, 2pt]{QED.}\\ \textbf{b. Case I }m=n \Rightarrow \int_{-\pi}^\pi \sin mx \sin nx\,dx =\int_{-\pi}^\pi \sin^2 nx \,dx= {1\over 2}\int_{-\pi}^\pi \left( 1-\cos 2nx\right)\,dx \\\qquad ={1\over 2}\left( \left. \left[ x-{1\over 2n} \sin 2nx \right] \right|_{-\pi}^\pi\right) ={1\over 2}\cdot 2\pi=\pi\\ \textbf{Case II }m\ne n\Rightarrow \int_{-\pi}^\pi \sin mx \sin nx\,dx =-{1\over 2} \int_{-\pi}^\pi\left( \cos(m+n)x- \cos(m-n)x\right)\,dx \\\qquad =-{1\over 2} \left( \left. \left[ {1\over m+n} \sin(m+n)x -{1\over m-n}\sin(m-n)x \right] \right|_{-\pi}^\pi \right) =-{1\over 2}\cdot 0=0 \;\bbox[red,2pt]{QED.}$$
解答:$$\cases{x(t) =5\cos t-\cos 5t\\ y(t)= 5\sin t-\sin 5t} \Rightarrow \cases{x'(t) = -5\sin t+5\sin 5t\\ y'(t) =5\cos t-5\cos 5t} \\ \Rightarrow \text{ length of the curve }=\int_0^{\pi/2} \sqrt{x'(t)^2 +y'(t)^2} \,dt =\int_0^{\pi/2} \sqrt{50-50(\cos t\cos 5t+ \sin t\sin 5t)} \,dt \\= \int_0^{\pi/2} \sqrt{50-50 \cos 4t}\,dt= \int_0^{\pi/2} \sqrt{50-50 (2\cos^2 2t-1)}\,dt = \int_0^{\pi/2} \sqrt{100-100 \cos^2 2t }\,dt \\=\int_0^{\pi/2}10\sqrt{1-\cos^2 2t}\,dt =\int_0^{\pi/2} 10\sin 2t\,dt =\left. \left[ -5\cos 2t\right] \right|_0^{\pi/2} = \bbox[red, 2pt]{10}$$
解答:$$a_n= {(-3)^n x^n \over \sqrt{n+1}} \Rightarrow \lim_{n\to \infty} \left|{a_{n+1} \over a_n} \right| =\lim_{n\to \infty} \left|{(-3)^{n+1}x^{n+1} \over \sqrt{n+2}} \cdot { \sqrt{n+1} \over (-3)^n x^n}\right| = 3|x|\lt 1 \Rightarrow -{1\over 3}\lt x\lt {1\over 3} \\ x={1\over 3} \Rightarrow \sum_{n=1}^\infty a_n=\sum_{n=1}^\infty { (-1)^n\over \sqrt{n+1}} \text{ convergent (by alternating series test) } \\x=-{1\over 3} \Rightarrow \sum_{n=1}^\infty a_n=\sum_{n=1}^\infty {1\over \sqrt {n+1}} \Rightarrow \int_1^\infty {1\over \sqrt{1+x}}\,dx \to \infty \Rightarrow \text{divergent} \\ \Rightarrow \text{interval of convergence: } \bbox[red, 2pt]{(-{1\over 3}, {1\over 3}]}$$
解答:$$x^2+y^2 =2x \Rightarrow (x-1)^2+y^2=1 \Rightarrow D=\{(x,y) \mid (x-1)^2+y^2 \le 1\} \\ \Rightarrow V= \iint_D z\,dA = \iint_D (x^2+y^2)\,dA \\ \text{By polar coordinate, }\cases{x=r\cos \theta\\ y= r\sin \theta} \Rightarrow D=\{ (r,\theta) \mid -{\pi\over 2}\le \theta \le {\pi\over 2}, 0\le r\le 2\cos \theta\} \\ \Rightarrow V=\iint_D r^2\cdot r\,drd\theta = \int_{-\pi/2}^{\pi/2} \int_0^{2\cos \theta}r^3 \,dr\,d\theta =4 \int_{-\pi/2}^{\pi/2} \cos^4 \theta\,d\theta =8\int_0^{\pi/2} \cos^4 \theta\, d\theta \\=8\int_0^{\pi/2} \left({\cos 2\theta+1\over 2} \right)^2\, d\theta =2 \int_0^{\pi/2}\left( 1+2\cos 2\theta + {\cos 4\theta+1\over 2} \right), d\theta= \bbox[red, 2pt]{3\pi\over 2}$$
解答:$$I= \int_{-2}^2 \int_{-\sqrt{4-x^2}}^{\sqrt{4-x^2}} \int_{\sqrt{x^2+y^2}}^2 (x^2+y^2)\,dzdy dx =\int_{-2}^2 \int_{-\sqrt{4-x^2}}^{\sqrt{4-x^2}} (x^2+y^2) (2-\sqrt{x^2+y^2}) \,dydx \\=\int_0^{2\pi} \int_0^2 r^2(2-r) r\,drd\theta =\int_0^{2\pi} \int_0^2 (2r^3-r^4)\,drd\theta = \int_0^{2\pi} {8\over 5}\,d\theta= \bbox[red, 2pt]{16\pi\over 5}$$
======================= END ======================
解題僅供參考,轉學考歷年試題及詳解

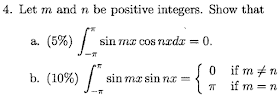
沒有留言:
張貼留言