110年公務人員高等考試二級考試試題
類 科: 工業行政
科 目: 統計學
解答:$$H_0:2010年與2021年家庭型態的分配沒有差異\\2021年抽樣戶數=412+ 128+209+55 = 804\\\begin{array}{} 型態&2010年比例(\%) \;& 2021年觀察值O_i \;& 2021年期望值(E_i)\\\hline 核心家戶& 54.3&412& 804\times 54.3\%= 436 \\ 主幹家戶 & 16.4 & 128& 804\times 16.4\%= 132 \\ 單人家戶& 22.0 & 209 & 804 \times 22\% = 177 \\ 其他家戶 & 7.3& 55 & 804 \times 7.3\%= 59 \\\hline \end{array} \\ \Rightarrow \chi^2 ={(412-436)^2 \over 436} +{(128-132)^2\over 132} +{(209-177)^2 \over 177} +{(55-59)^2\over 59} = 7.5\\ 自由度df=(4-1)\times(2-1)=3 \Rightarrow 查試卷附表可知\chi^2(df=3,\alpha=0.05)=7.815\\ \Rightarrow \chi^2 \not \gt \chi^2(3,0.05) \Rightarrow \bbox[red,2pt]{不能拒絕H_0},即兩年的家庭分配\bbox[red,2pt]{沒有變化}$$
解答:
(一)$$\begin{array}{cc|ccc} & & & Y & \\ & & 0 & 1 & 2\\\hline & 0 & 0 & 60/600=0.1 & 18/600=0.03 \\X & 1 & 240/600=0.4& 90/600 =0.15 & 30/600= 0.05\\ & 2 & 120/600=0.2 & 30/600=0.05 & 12 /600=0.02\\\hline\end{array}\\ \Rightarrow X與Y的聯合機率分配為\\\bbox[red,2pt]{ P(X=0,Y=0)=0、P(X=0,Y=1)=0.1、P(X=0,Y=2)=0.03\\P(X=1,Y=0)=0.4、P(X=1,Y=1)=0.15、P(X=1,Y=2)=0.05\\ P(X=2,Y=0)=0.2、P(X=2,Y=1)=0.05、P(X=2,Y=2)=0.02\quad}$$(二)$$由(一)可知: P(X=0)=0+0.1+0.03 =0.13、P(X=1)=0.4+0.15+0.05 =0.6\\、P(X=2 )=0.2+0.05+0.02 =0.27\\因此\cases{E(X)=0\cdot 0.13+1\cdot 0.6+ 2\cdot 0.27= 1.14\\ E(X^2) =0^2\cdot 0.13+1^2\cdot 0.6+ 2^2\cdot 0.27= 1.68} \\\Rightarrow Var(X)=E(X^2)-(E(X))^2 =1.68-1.14^2 =0.3804 \Rightarrow \bbox[red,2pt]{\cases{期望值E(X)=1.14\\ 變異數Var(X)=0.3804}}$$(三)$$P(T=1)=P(X=0,Y=1)+ P(X=1,Y=0)=0.1+0.4=0.5 \\P(T=2)=P(X=0,Y=2)+P(X=Y=1) +P(X=2,Y=0)= 0.03+0.15+0.2 =0.38\\ P(T=3)= P(X=1,Y=2)+P(X=2,Y=1)= 0.05+0.05=0.1\\P(T=4) =P(X=Y=2)=0.02\\ \Rightarrow \cases{E(T)=1\cdot 0.5+2\cdot 0.38+ 3\cdot 0.1 + 4\cdot 0.02 = 1.64\\ E(T^2)= 1^2\cdot 0.5+2^2 \cdot 0.38+ 3^2\cdot 0.1 + 4^2\cdot 0.02 = 3.24} \\ \Rightarrow Var(T)=E(T^2)-(E(T))^2 =3.24-1.64^2 =0.5504 \\ \Rightarrow \bbox[red,2pt]{\cases{T的期望值=1.64\\ T的變異數=0.5504}}$$
解答:
(一)$$n= (t_{\alpha/2})^2{p(1-p)\over E^2} =t_{0.025}(\infty)\cdot {0.13\times (1-0.13) \over 0.02^2}=1.96\times 282.75 =554.19 \Rightarrow n=\bbox[red,2pt]{555}$$(二)$$p={109\over 555} \Rightarrow 信賴區間=p\pm t_{\alpha/2}\sqrt{p(1-p)\over n} ={109\over 555} \pm 2.576\cdot \sqrt{{109\over 555}\cdot {446\over 555}\over 555} =0.196\pm 0.043\\ =\bbox[red,2pt]{0.153\text{~}0.240}$$

解答:
(一)$$標準差未知之常態母體,信賴區間:\bar X\pm t_{\alpha/2}(n-1){s\over \sqrt n};\\先求樣本標準差s=\sqrt{\sum x_i^2-(\sum x_i)^2/n \over n-1} =\sqrt{972804-3116^2/10\over 9} =14.37\\,再查表t_{0.025}(9)=2.262 \Rightarrow 信賴區間={3116\over 10}\pm 2.262\times {14.37\over \sqrt{10}}=311.6\pm 10.279\\= \bbox[red,2pt]{301.321\text{~}321.879}$$
(二)$$\cases{H_0:\sigma^2=300\\ H_1:\sigma^2 \gt 300},現在\cases{n=10\\ df=n-1=9\\ s^2=14.37^2\\ \alpha=0.05} \Rightarrow \chi^2={(n-1)s^2\over \sigma^2} = {9\times 14.37^2\over 300} =6.195\\ 查表\chi^2(df=9,\alpha=0.05)=16.919 \Rightarrow \chi^2 \not \gt \chi^2(9,0.05) \Rightarrow 不能拒絕H_0,即母體變異數\bbox[red,2pt]{不大於300}$$









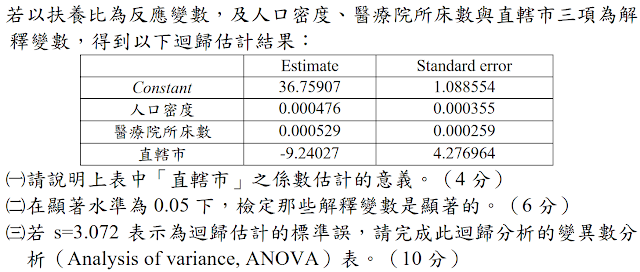

沒有留言:
張貼留言