103 年公務人員初等考試
解答:$$溫度可比較高低(加減運算),不能計算比率,故選\bbox[red,2pt]{(C)}$$
解答:$$不同單位,其平均數不同,因此需採用CV而非VAR,故選\bbox[red,2pt]{(D)}$$
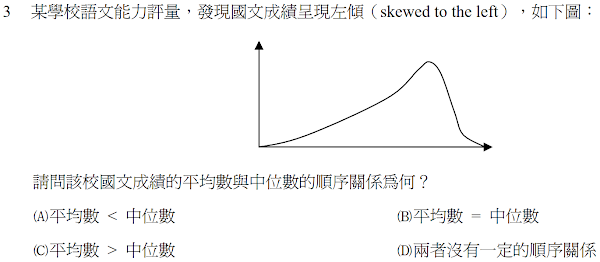
解答:$$圖形為左偏,因此中位數\gt 平均數,故選\bbox[red,2pt]{(A)}$$
解答:$$P(1,1)+P(1,2)+ P(2,1) + P(1,3)+ P(3,1)+ P(2,2) \\=({1\over 4})^2+{1\over 4}\cdot {1\over 8}+{1\over 8}\cdot {1\over 4}+{1\over 4}\cdot {1\over 8} +{1\over 8}\cdot {1\over 4}+ ({1\over 8})^2 \\ ={1\over 16}+ {4\over 32} +{1\over 64} ={ 13\over 64},故選\bbox[red,2pt]{(C)}$$
解答:$${檢驗為陰性且無患病\over 檢驗為陰性且患病+檢驗為陰性且無患病} ={95\% \times 8599\%\over 5\% \times 12\% + 95\%\times 85\%} = 99.262\%,故選\bbox[red,2pt]{(D)}$$
解答:
$$\cases{A+D+F+G = 220\\ B+D+E +G=180\\ C+E+F+G= 150\\ D+G = 150\\ F+G=80\\ E+G=100\\ A+B+C+D +E+F+G=300} \Rightarrow \cases{A+F=70\\ B+D=80\\ C+E=70} \Rightarrow A+B+C+D+E+F =220\\ \Rightarrow G=300-220=80 \Rightarrow 機率={80\over 300}={4\over 15},故選\bbox[red,2pt]{(D)}$$
解答:$$P(A^c\cap B^c) = 1-P(A\cup B)=1-(P(A)+P(B)- P(A\cap B))= 1-(0.3+0.4-0.3\cdot 0.4)\\ =0.42,故選\bbox[red,2pt]{(A)}$$
解答:$$\cases{P(A\mid B)=P(A\cap B)/P(B)=0.25 \\ P(B\mid A)=P(A\cap B)/P(A) =0.5} \Rightarrow P(A \cap B)=0.25P(B)= 0.5P(A) \\ \Rightarrow P(A)= 0.25P(B)/0.5 = 0.25\times 0.3\div 0.5=0.15,故選\bbox[red,2pt]{(D)}$$
解答:$$X\sim B(n=20,p=180/300) \Rightarrow Var(X)=np(1-p)=20\times {3\over 5}\times{2\over 5}=4.8\\由於有限母體,需校正,即4.8\times {N-n\over N-1}=4.8\times {280\over 299}=4.495,故選\bbox[red,2pt]{(C)}$$
解答:$$當N\to \infty時,超幾何趨近二項分配,故選\bbox[red,2pt]{(A)}$$
解答:$$指數分配,其E(Y)=1/43,故選\bbox[red,2pt]{(D)}$$
解答:$$\cases{E(X)=\lambda\\ E(Y)=1/\lambda} \Rightarrow E(X)E(Y)=1,故選\bbox[red,2pt]{(D)}$$
解答:$$z分配,故選\bbox[red,2pt]{(C)}$$
解答:$$P(X\ge 2)=1-P(X=0)-P(X=1)= 1-0.6^{10}-C^{10}_1\cdot 0.6^9\cdot 0.4 =0.9536,故選\bbox[red,2pt]{(D)}$$
解答:$$\cases{男生上網比例p_1=310/560\\ 女生上網比例p_2=300/640} \Rightarrow \sqrt{{p_1(1-p_1)\over 560} +{p_2(1-p_2)\over 640}} =0.02882,故選\bbox[red,2pt]{(B)}$$
解答:$$E(\hat \theta-\theta)=E(\hat \theta)-\theta,故選\bbox[red,2pt]{(C)}$$
解答:$$\cases{母體的一階動差=E(X)={0+\theta\over 2} ={\theta\over 2}\\ 樣本的一階動差={1\over n}(X_1+\cdots +X_n)=\bar X}\\ \Rightarrow 兩者相等,即{\theta \over 2}=\bar X \Rightarrow \theta =2\bar X,故選\bbox[red,2pt]{(C)}$$
解答:$$n\ge z_{\alpha/2}\cdot {p(1-p)\over E^2} =1.96^2 \times {0.3\times 0.7\over 0.03^2} =896.37 \Rightarrow n=897,故選\bbox[red,2pt]{(B)}$$
解答:$$E({\sum X_i^2\over n}) ={1\over n}E(X_1^2 +X_2^2 +\cdots +X_n^2) ={1\over n}\left( n(\sigma^2 +\mu^2)\right) =\sigma^2 +\mu^2 \\ \Rightarrow {\sum X_i^2\over n} 是\sigma^2 +\mu^2的不偏估計量\\ E({\sum (X_i-\bar X)^2\over n}) ={1\over n} E(\sum X_i^2-n(\bar X)^2) ={1\over n}\left( \sum E(X^2)-nE((\bar X)^2)\right)\\ ={1\over n}\left( n(\sigma^2+\mu^2) -n\left({\sigma^2\over n}+\mu^2 \right)\right) ={n-1\over n} \sigma^2 \ne \sigma^2\\ \Rightarrow {\sum (X_i-\bar X)^2\over n} 不是\sigma^2的不偏估計量\\,故選\bbox[red,2pt]{(B)}$$
解答:$$z_{0.025}/z_{0.05} =1.96/1.65=1.1879,故選\bbox[red,2pt]{(C)}$$
解答:$$p值\lt \alpha,則拒絕H_0,故選\bbox[red,2pt]{(B)}$$
解答:$$樣本數增加,則誤差減少,故選\bbox[red,2pt]{(B)}$$
解答:$$\cases{H_0:p\le 0.3\\ H_1:p\gt 0.3},若能拒絕H_0則證明p\gt 30\%是正確的,因此對立假設為p\gt 0.3,故選\bbox[red,2pt]{(D)}$$
解答:$$\alpha越大,拒絕H_0機會越大,接受H_0機率越小,因此\beta變小,故選\bbox[red,2pt]{(B)}$$
解答:$$0.006 小於 1\%、5\%,也小於1\%/2、5\%/2;且0.006 \gt 0.1\%,故選\bbox[red,2pt]{(B)}$$
解答:$${600-\bar x\over \sqrt{50/20}} \gt z_{0.05} =1.645 \Rightarrow \bar x\lt 600-1.645\times \sqrt{2.5}=597.399,故選\bbox[red,2pt]{(D)}$$
解答:$$各組相同,即各組平均數相同,故選\bbox[red,2pt]{(D)}$$
解答:$$ANOVA檢定統計量為F,故選\bbox[red,2pt]{(B)}$$
解答:$$共k組,因此自由度為k-1,故選\bbox[red,2pt]{(B)}$$
解答:$$k個\alpha_i及1個\mu,共k+1個參數,故選\bbox[red,2pt]{(B)}$$
解答:$$相關係數r=1.5916\times {8.88\over 14.45} =0.97809,故選\bbox[red,2pt]{(D)}$$
解答:$$E\hat \theta_1 = E\hat\theta_2 = \theta \Rightarrow \hat \theta_1與\hat \theta_2 為不偏估計量,故選\bbox[red,2pt]{(D)}$$
解答:$$(5-1)\times (8-1)=28,故選\bbox[red,2pt]{(C)}$$
解答:$$每個細格需\ge 5,故選\bbox[red,2pt]{(C)}$$
解答:$$10組\Rightarrow 自由度=10-1=9,再加上適合度檢定,因此9-1=8,故選\bbox[red,2pt]{(B)}$$
解答:$$\cases{總反對人數=55+30+25 = 110,占比為{110\over 200}\\ 中部總人數=20+30=50} \Rightarrow 中部反對預期人數=50\times {110\over 200} =27.5\\,故選\bbox[red,2pt]{(A)}$$
解答:$$期望值:\begin{array}{|c|c|c|c|}\hline & 北部 & 中部 & 南部\\\hline 贊成& 40.5 & 22.5 & 27 \\\hline 反對& 49.5 & 27.5 & 33\\\hline \end{array} \\ \Rightarrow 檢定統計量 = {(35-40.5)^2 \over 40.5} +{(20-22.5)^2 \over 22.5} +{(35-27)^2 \over 27} +{55-49.5)^2\over 49.5} +{(30-27.5)^2 \over 27.5}\\\qquad +{(25-33)^2\over 33}=6.1728,故選\bbox[red,2pt]{(D)}$$
解答:$$另一個是隨機,不是規則變動,故選\bbox[red,2pt]{(D)}$$
解答:$$評審有三位,自由度為3-1=2,故選\bbox[red,2pt]{(B)}$$
解答:$$\cases{F(10)=48.536\\ D(10)=48.7} \Rightarrow F(11)=F(10)+\alpha (D(10)-F(10)) \\ \Rightarrow F(11)=48.536+0.4(48.7-48.536) = 48.6016,故選\bbox[red,2pt]{(A)}$$
=================== END ===========================







































沒有留言:
張貼留言